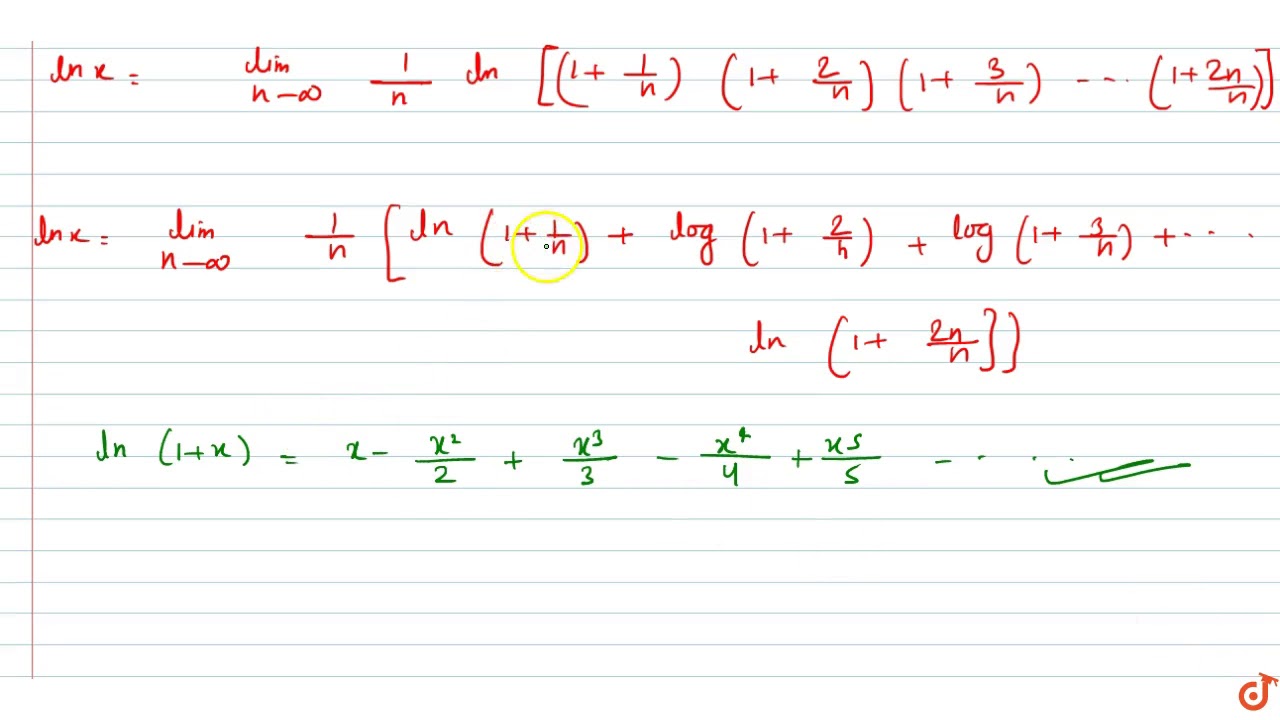1 2 1 4 1 8 Dots N 1 2n 1 1 2 N

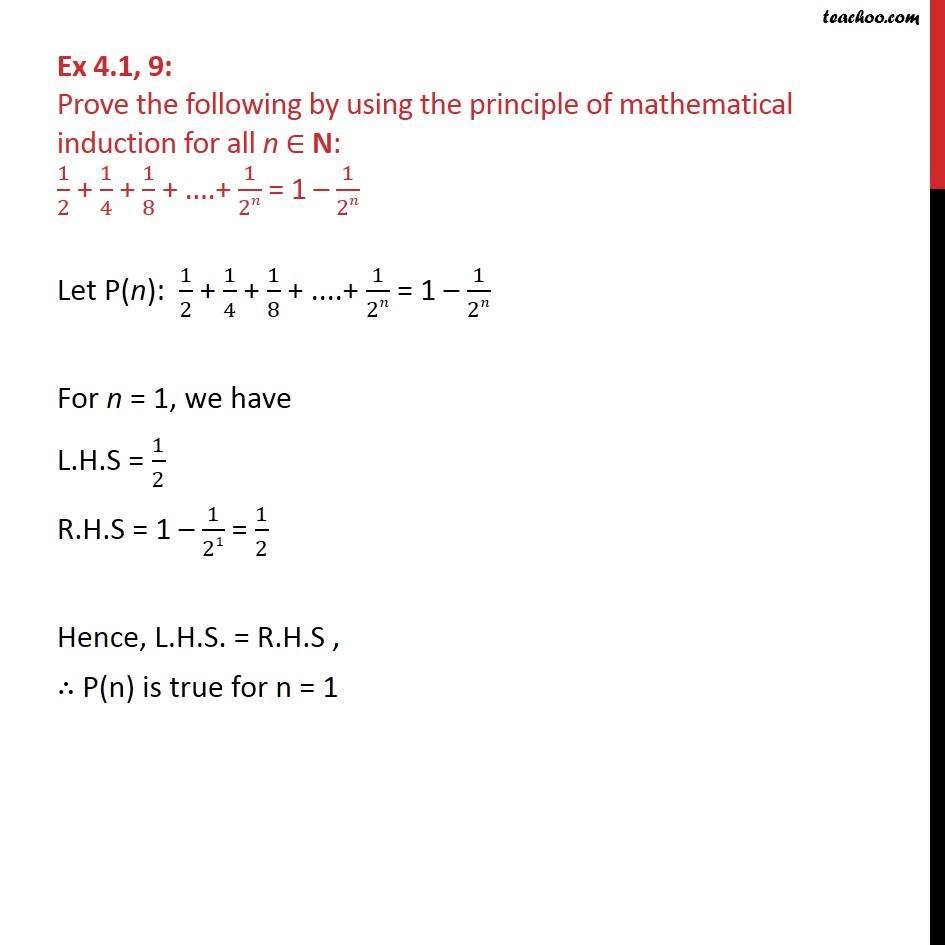
1 2 1 4 1 8 1 2𝑛 1 1 2𝑛 let p n.

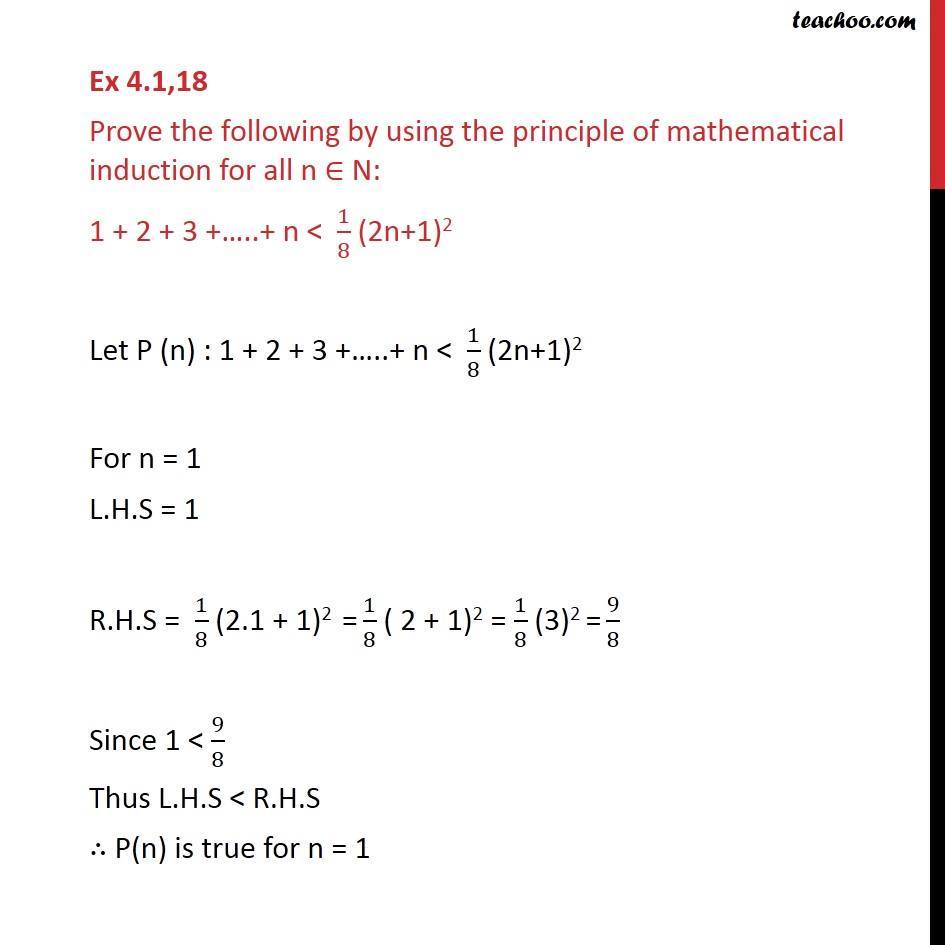
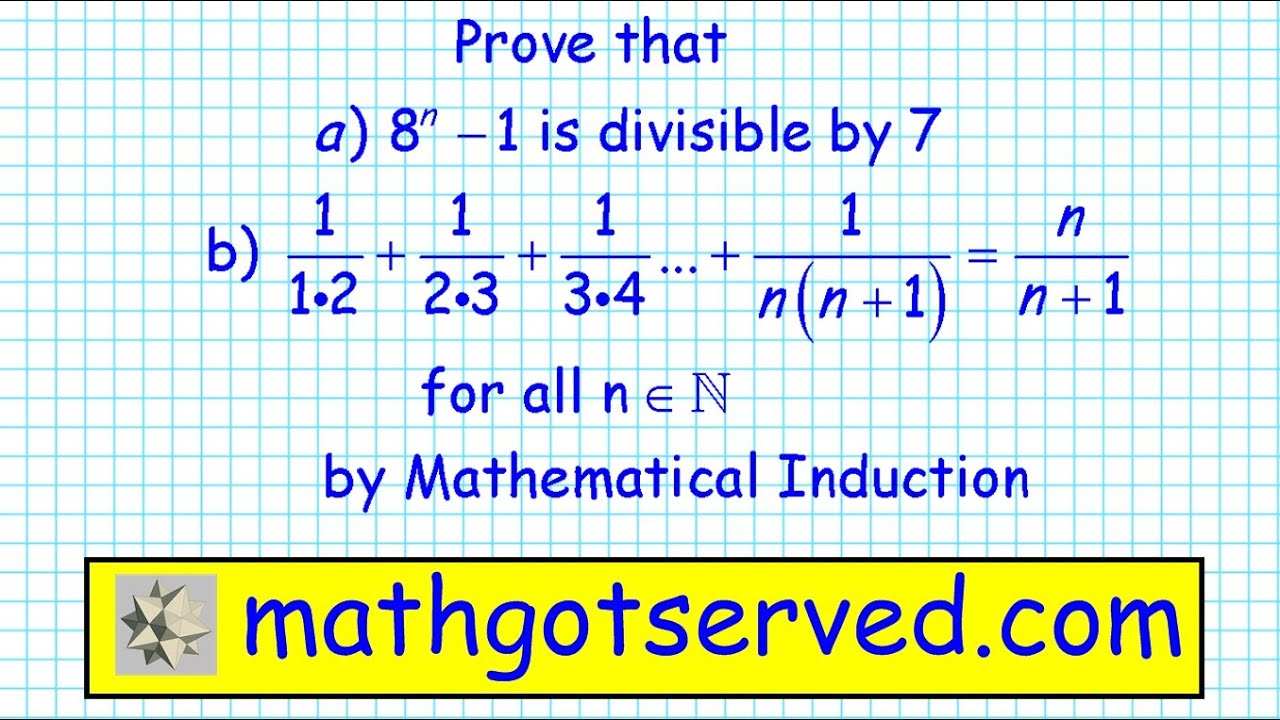
1 2 1 4 1 8 dots n 1 2n 1 1 2 n. N n 1 2 1 3 6 10 15 clearly the required sequence is double the one we have found the nth term for therefore the nth term of the required sequence is 2n n 1 2 n n 1. Rules like that are called recursive formulas. Prove the following by using the principle of mathematical induction for all n n. A monotonic path is one which starts in the lower left corner finishes in the upper right corner and consists entirely of edges pointing rightwards or upwards.

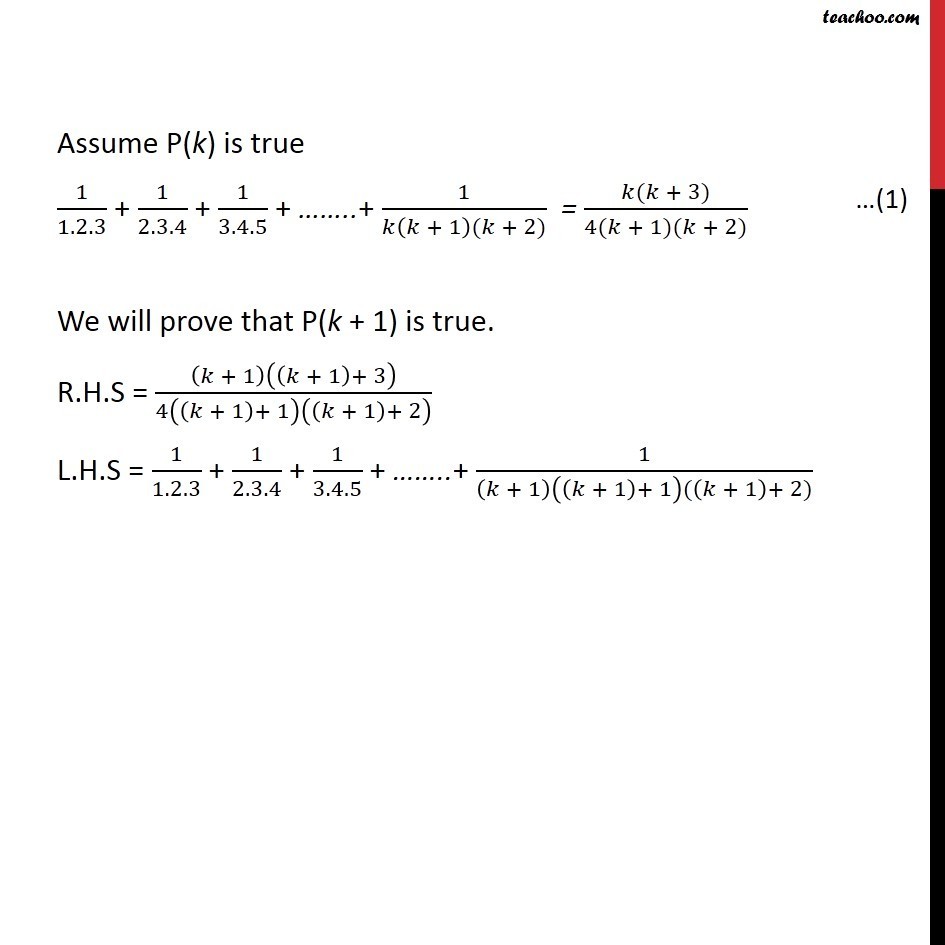
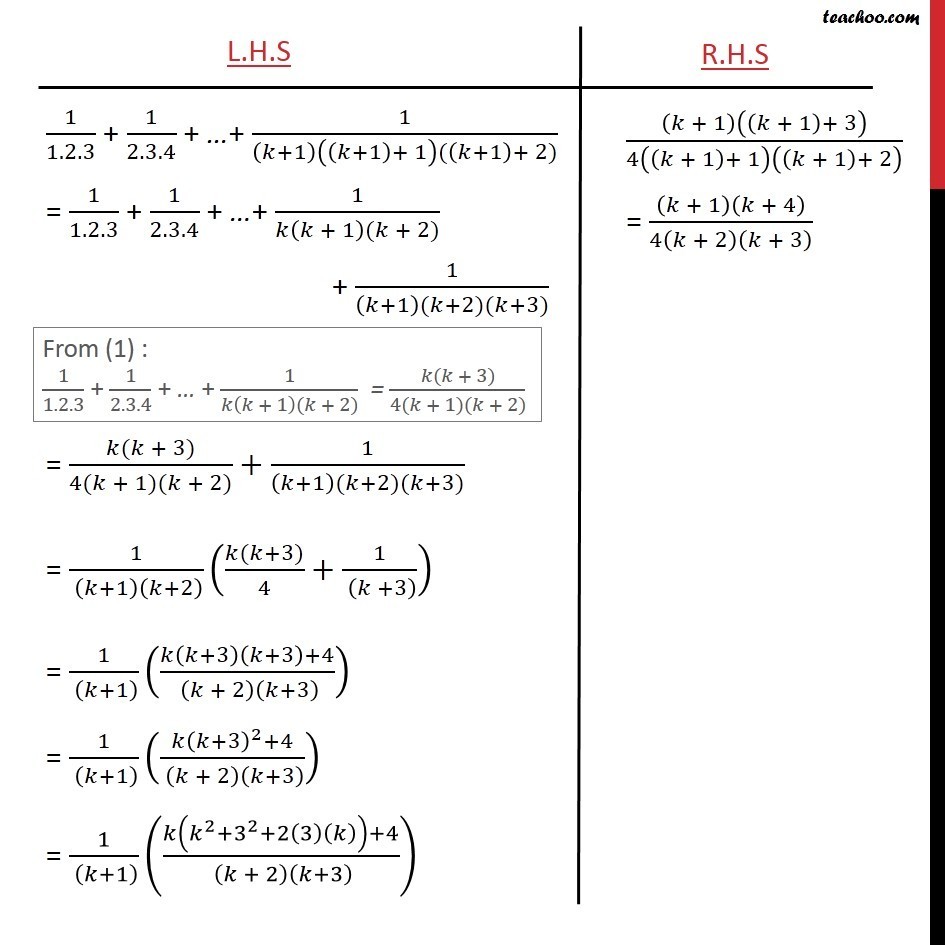
R h s p n is true for n 1 assume p k is true 1 2 1 4 1 8 1 2𝑘 1 1 2𝑘 we. C n is the number of monotonic lattice paths along the edges of a grid with n n square cells which do not pass above the diagonal. Rule is x n x n 1 x n 2 that rule is interesting because it depends on the values of the previous two terms. 1 2 1 4 1 8 1 2𝑛 1 1 2𝑛 for n 1 we have l h s 1 2 r h s 1 1 21 1 2 hence l h s.

Supercharge your algebraic intuition and problem solving skills. In mathematics the infinite series 1 2 1 4 1 8 1 16 is an elementary example of a geometric series that converges absolutely.