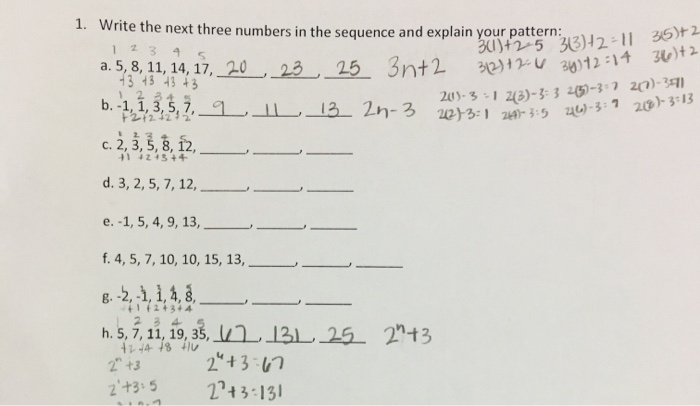1 1 2 3 5 8 Pattern

X 1 x 2 x 3 x 4 x 5 x 6 x 7. 4 2 2 2 2 1 1 2 1 2 1 2 2 1 4 1 4 2 1 8 and so on. Find more mathematics widgets in wolfram alpha.

apple watch series 4 golden apple watch series 4 44mm stainless steel black apple watch series 5 40mm nike edition apple watch series 3 nike sport band apple watch series 3 silver aluminum white sport band apple watch series 4 40mm vs 44mm apple watch series 4 44mm cellular apple watch series 5 40mm nike band

What Is The Pattern Of Sequence 1 1 2 3 5 8 13 Quora

Number Series And Puzzles Tricks And Solutions 1 1 2 3 5 8 13 Youtube

Https Encrypted Tbn0 Gstatic Com Images Q Tbn 3aand9gcttknk66fhx Bgym1ipo30agmadbg5xoayscq Usqp Cau

Fibonacci S Rainbow Dreamhouse Album On Imgur

The Fibonacci Sequence Nanotedsla

$The Fibonacci Sequence Nanotedsla$

Get the free pattern finder widget for your website blog wordpress blogger or igoogle.

1 1 2 3 5 8 pattern. The common ratio can be less than 1. In the present form of the gleason system prostate cancer of gleason patterns 1 and 2 are rarely seen. The fibonacci sequence is the series of integers. We can often describe number patterns in more than one way.

C program to print pyramids and patterns in this example you will learn to print half pyramids inverted pyramids full pyramids inverted full pyramids pascal s triangle and floyd s triangle in c programming. Look at this series. In the first pattern 3 is added. And the terms after the first term are obtained by adding 2 to the previous term.

To illustrate this consider the following sequence of numbers 1 3 5 7 9. Clearly the first term of this number pattern is 1. View answer discuss in forum workspace report. If we start with two small squares of size 1 next to each other.

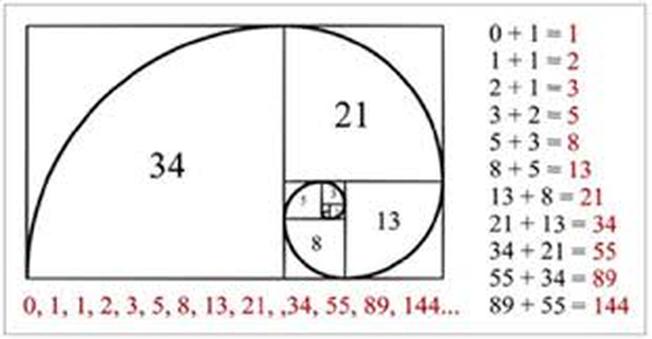
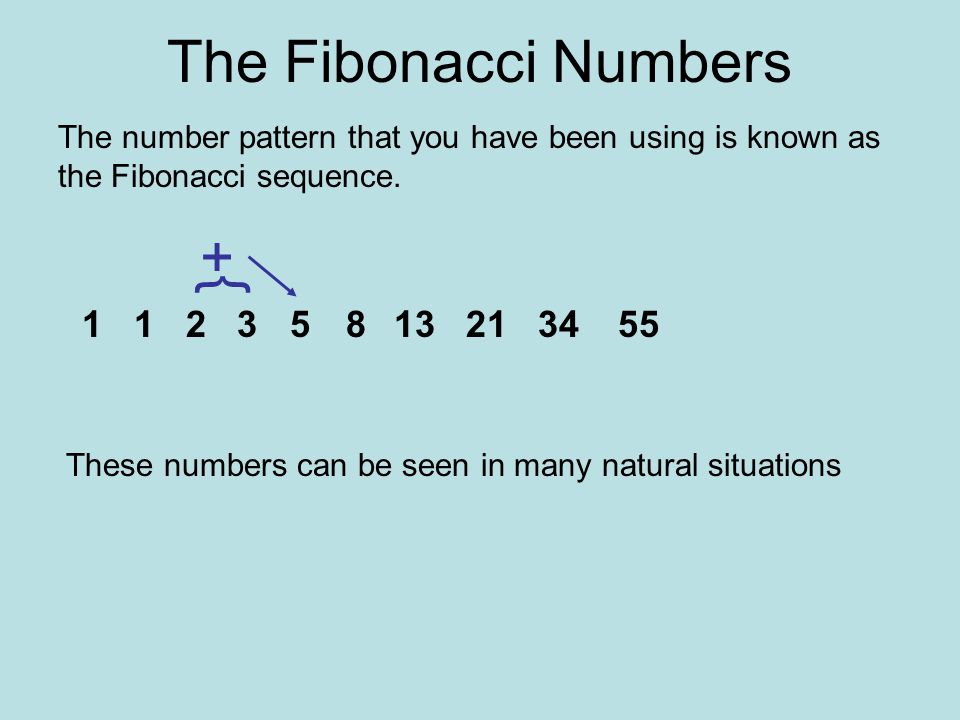
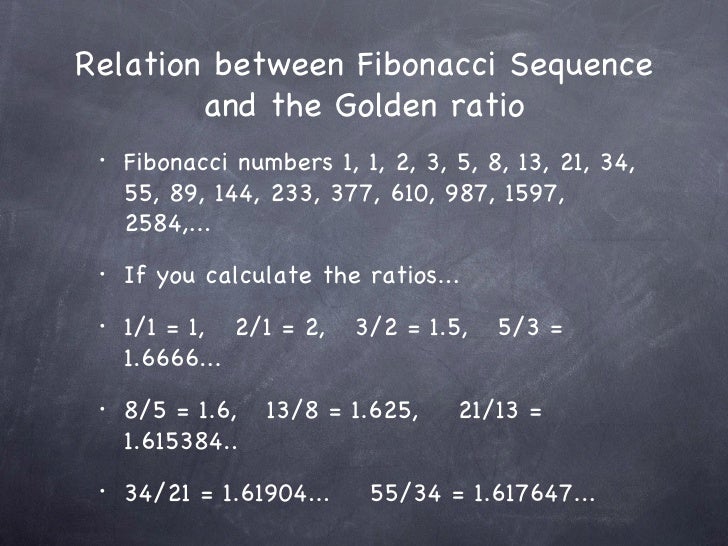
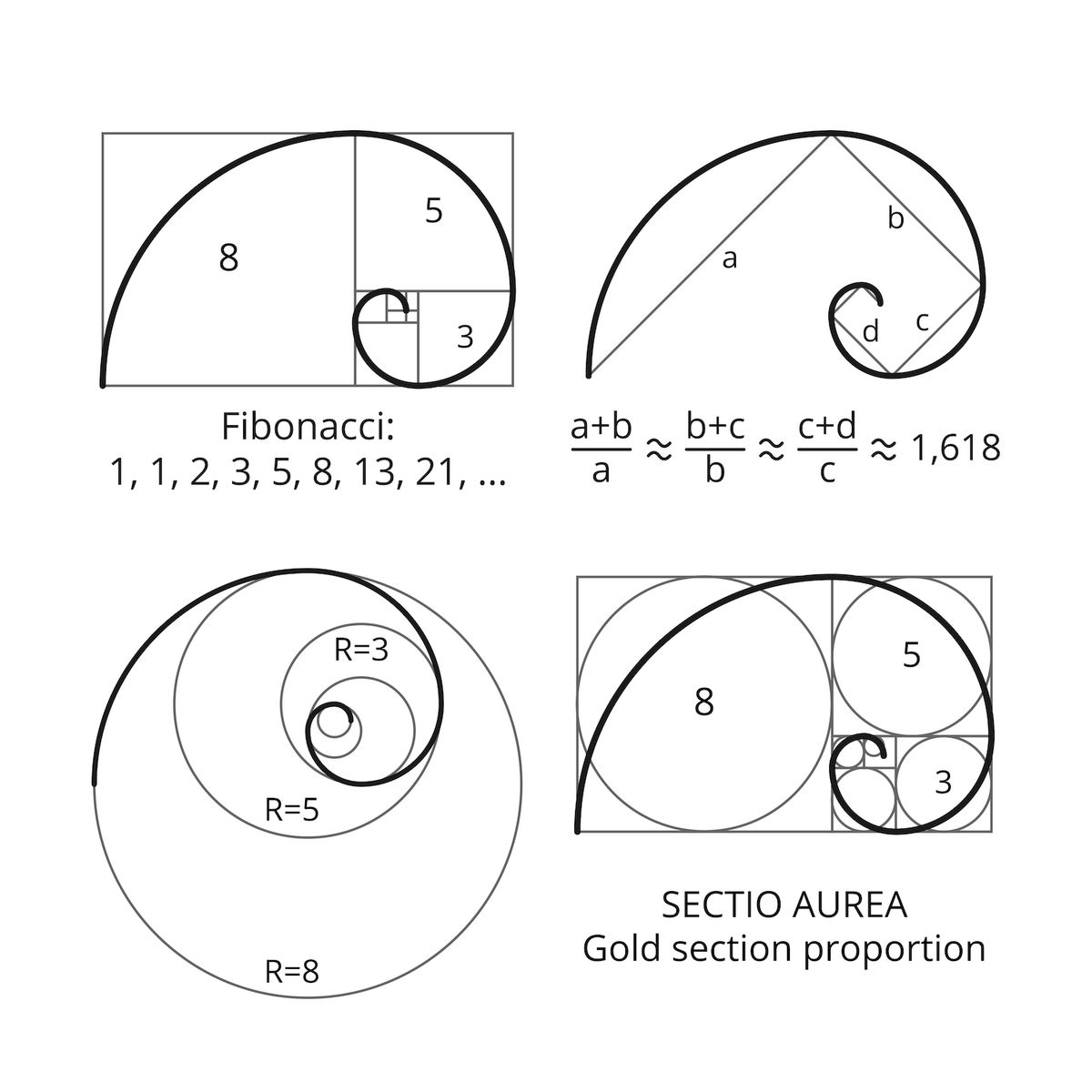
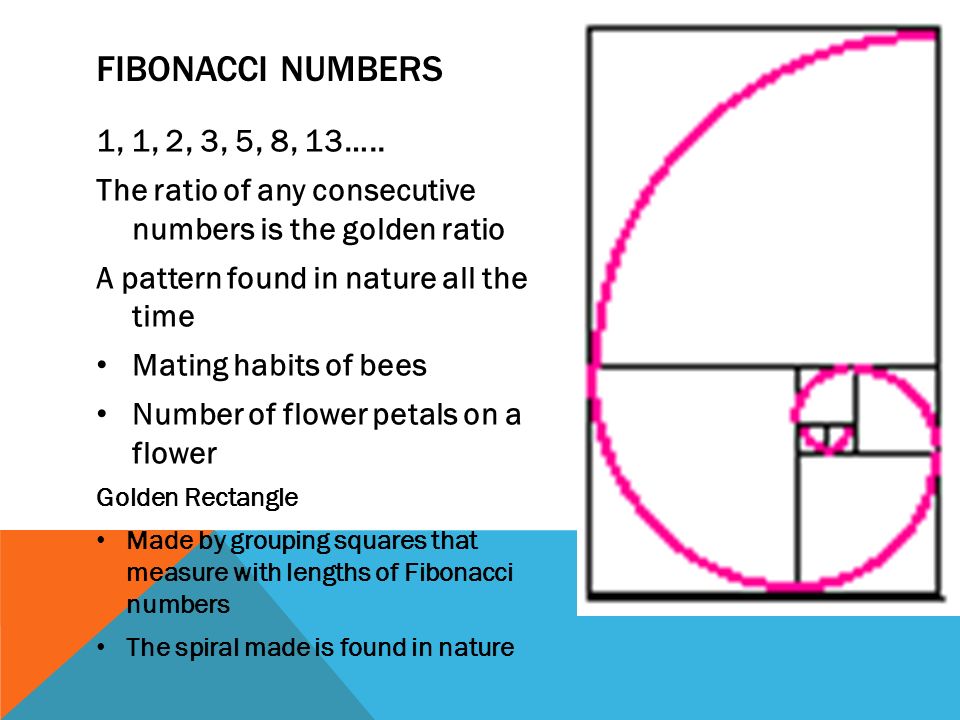
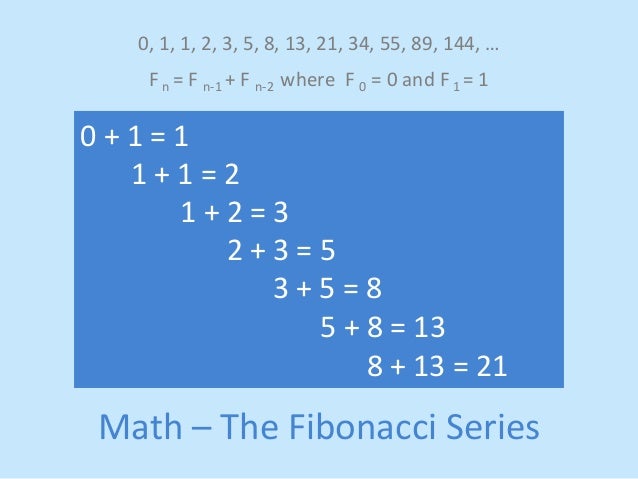
Each element in the series is the sum of the. We can make another picture showing the fibonacci numbers 1 1 2 3 5 8 13 21. Gleason pattern 3 is by far the most common. And then another touching both the 2 square and the 3 square which has.

The next number is found by adding up the two numbers before it. This corresponds to an anaplastic carcinoma. Pattern 5 the tissue does not have any or only a few recognizable glands. 1 2 2 4 2 8 2 16 2 2 2 pattern.

Report errors view. There are often just sheets of cells throughout the surrounding tissue. 0 1 1 2 3 5 8 13 21 34. The fibonacci sequence is the series of numbers.

This is a simple alternating addition and subtraction series. 0 1 1 2 3 5 8 13 21 34 55 89. 10 5 2 5 1 25 0 625. This sequence starts at 1 and has a common ratio of 2.

The pattern is continued by multiplying by 2 each time like this. In the second 2 is subtracted. Multiply the previous number by 2 to get the next one the dots at the end simply mean that the sequence can go on forever. 36 34 30 28.

We can now draw a new square touching both a unit square and the latest square of side 2 so having sides 3 units long. On top of both of these draw a square of size 2 1 1. When referring to sequences like this in mathematics we often represent every term by a special variable.