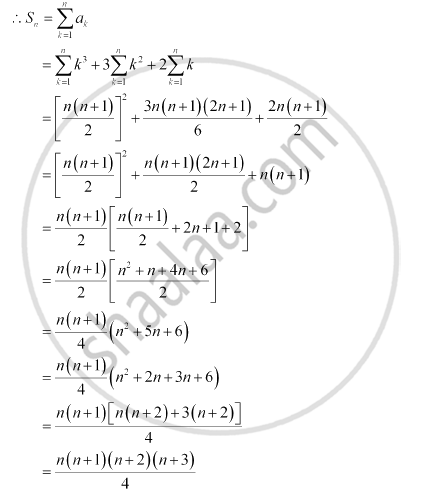1 1 1 2 1 N

Its name derives from the concept of overtones or harmonics in music.

1 1 1 2 1 n. Following is the implementation of simple solution. 1 a n n 1 n 1. 2 2. Given a positive integer n write a function to compute sum of the series 1 1.

I can give you a good approximation if you would prefer. If inverse of a sequence follows rule of an a p i e arithmetic progression then it is said to be in harmonic progression in general the terms in a harmonic progression can be denoted as. Means n factorial or n n 1 n 2. In mathematics the harmonic series is the divergent infinite series.

3 3. What is the value of 1 1. A simple solution solution is to initialize sum as 0 then run a loop and call factorial function inside the loop. Click here to get an answer to your question 1 2 1 1 n 3 1 1 n 2 terms.

1 a 1 a d 1 a 2d 1 a 3d.